Nonlinear vibration-based identification of delamination. Experimental investigations and numerical simulation of delaminated structures
- contact: Prof. Dr.-Ing. Peter Vielsack
Dr.-Ing. Ingolf Müller
Dr.-Ing. Hans Schmieg - funding: Deutsche Forschungsgemeinschaft (DFG)
- Detection and localisation of delaminations on the basis of vibration behaviour
- Experimental investigations and numerical simulation of delaminated structures
Description of the project
VIBRATION-BASED IDENTIFICATION OF DELAMINATIONS
Sandwich and laminate materials are being used increasingly in engineering applications due to their high specific stiffness and strength. The presence of damage, in particular delaminations between adjacent plies, may severely degrade the mechanical properties of a structure. Therefore, the ability of non-destructive testing and monitoring of the structural integrity becomes an important issue.
Within the project a vibration-based approach for the identification of delaminations is considered. The investigations are extended to the experimental investigation of the vibration behaviour and the numerical modelling for model-based identification techniques. Unilateral constraints and dissipative impacts along the damaged region dominate the oscillations of delaminated structures. These phenomena give rise to strong nonlinear system response, whereas the degree of the nonlinearity augments with increasing intensity of excitation.
The numerical modelling leads due to the strong nonlinearities to the
consideration of a non-smooth dynamical system. An appropriate description for the dissipative contact dominating the vibro-impacting system is of a great importance for the accurate capture of motion. Depending on the systems properties as well as the amplitude and the frequency of a harmonic excitation a bifurcation scenario up to chaotic motion may occur.
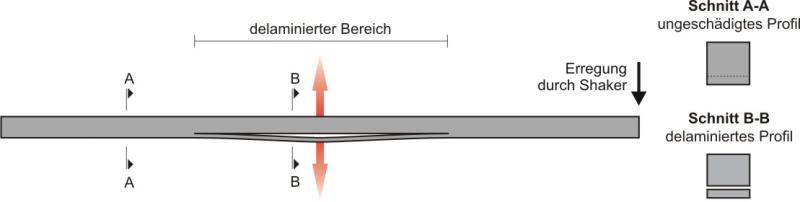
Figure 1: Example of a delaminated beam
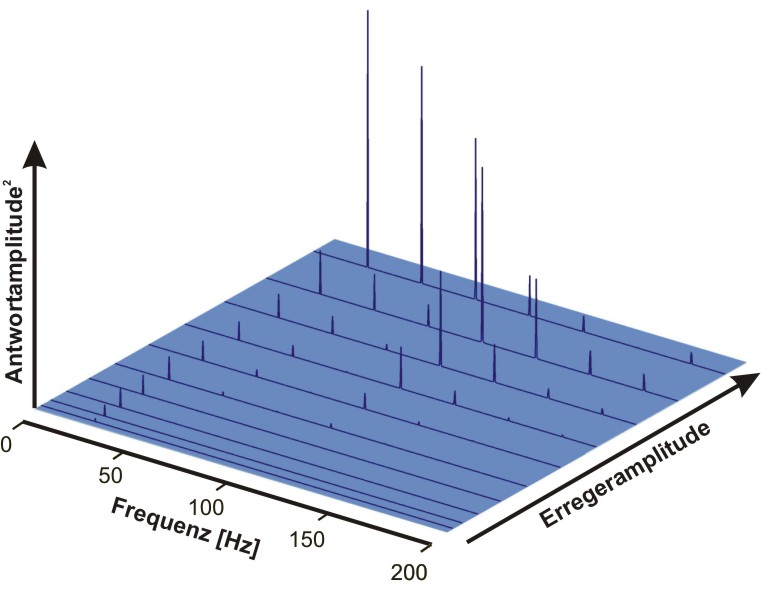
Figure 2: Augmentation of the nonlinearity of the system's response by
increase of amplitude of the harmonic excitation.

Figure 3: Distribution of the nonlinearity of the system's response for an experimental identification of delamination.
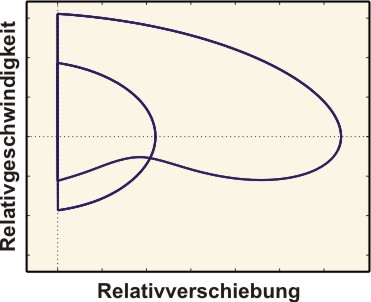
Figure 4: Phase portrait for the relative motion of delaminated layer and the remaining beam with one impact-like contact phase in
one excitation period.
